Are you curious to know what is a coterminal angle? You have come to the right place as I am going to tell you everything about a coterminal angle in a very simple explanation. Without further discussion let’s begin to know what is a coterminal angle?
In the realm of trigonometry, the concept of coterminal angles plays a crucial role in understanding the measurement of angles and their relationships. Let’s embark on a journey to explore what coterminal angles are, how to find them, and their significance in trigonometric calculations.
What Is A Coterminal Angle?
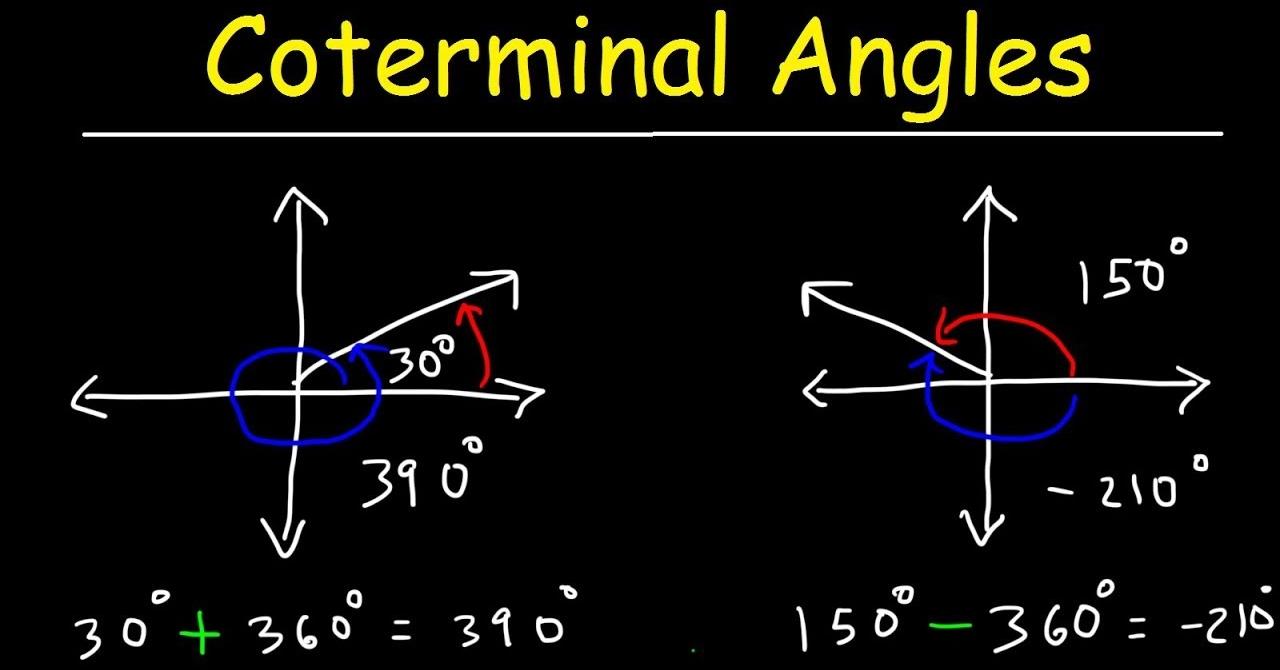
A coterminal angle is an angle that, when added or subtracted by a multiple of 360 degrees (or 2π radians), results in the same position on the unit circle. In simpler terms, coterminal angles share the same initial and terminal sides, forming a coexisting position in the coordinate plane.
What Is A Coterminal Angle In Trigonometry?
In trigonometry, coterminal angles are pivotal for simplifying calculations and understanding periodic functions. They allow mathematicians to work with angles beyond a single revolution, providing a comprehensive view of angle measurements on the unit circle.
How To Find Coterminal Angles:
To find coterminal angles, one can add or subtract multiples of 360 degrees or 2π radians to the given angle. The resulting angles will have the same terminal side on the unit circle. For example, if θ is an angle, then θ ± 360°, θ ± 720°, and so on, are coterminal angles.
What Is A Coterminal Angle Example:
Consider an angle θ = 45°. Its coterminal angles include 45° + 360° = 405°, 45° – 360° = -315°, and so forth. These angles share the same position on the unit circle, despite the variations in their measures.
How To Find Coterminal Angles In Radians:
Similarly, in radians, one can find coterminal angles by adding or subtracting multiples of 2π. If θ is an angle measured in radians, then θ ± 2π, θ ± 4π, and so on, represent coterminal angles. The key is to maintain the same terminal side on the unit circle.
What Is A Coterminal Angle Of 20 Times Pi Over 3?
To find the coterminal angle of 20 times π over 3, one can add or subtract multiples of 2π. The coterminal angles will be 20π/3 + 2πk, where k is an integer. This formula generates an infinite set of coterminal angles that share the same position on the unit circle.
Coterminal Angle Calculator:
For convenience, coterminal angle calculators are available online. These tools facilitate quick calculations by generating coterminal angles based on user-inputted values. However, understanding the manual process remains crucial for a comprehensive grasp of the concept.
Visit Singerbio and read everything about the singer.
Negative Coterminal Angle:
A negative coterminal angle is obtained by subtracting multiples of 360 degrees or 2π radians from the given angle. For example, if θ = 120°, then its negative coterminal angle is 120° – 360° = -240°.
Coterminal Meaning:
The term “coterminal” originates from the Latin word “co-” meaning together and “terminalis” meaning boundary. In the context of angles, coterminal angles share the same terminal side or boundary on the unit circle.
Coterminal And Reference Angles:
Coterminal angles and reference angles are interconnected concepts. While coterminal angles share the same terminal side, reference angles measure the acute angle formed by the terminal side and the x-axis. Reference angles are often used in trigonometric calculations.
Conclusion:
In conclusion, coterminal angles are a fundamental concept in trigonometry, providing a way to represent angles beyond a single revolution. Understanding how to find coterminal angles empowers mathematicians to navigate the complexities of angle measurements, offering a comprehensive view of trigonometric functions and periodicity. So, the next time you encounter coterminal angles in trigonometric calculations, appreciate their role in simplifying mathematical concepts and enhancing your grasp of angle relationships.
FAQ
What Is The Definition Of Coterminal Angles?
Coterminal angles are angles in standard position (angles with the initial side on the positive x -axis) that have a common terminal side. For example 30° , −330° and 390° are all coterminal.
What Is A Coterminal Angle To 45?
In Mathematics, the coterminal angle is defined as an angle, where two angles are drawn in the standard position. Also both have their terminal sides in the same location. For example, the coterminal angle of 45 is 405 and -315.
Is Coterminal 180 Or 360?
Coterminal angles have the same initial and terminal sides. The simplest case is 180°. If you imagine this on a cartesian plane, it is simply the x-axis. The 180° on the positive y-axis side is coterminal with the 180° on the negative y-axis side and vice versa.
What Is The Coterminal Angle Of 400?
Find an angle that is positive, less than 360° , and coterminal with 400° . Subtract 360° 360 ° from 400° 400 ° . The resulting angle of 40° 40 ° is positive, less than 360° 360 ° , and coterminal with 400° 400 ° .
I Have Covered All The Following Queries And Topics In The Above Article
What Is A Coterminal Angle In Trig
What Is A Coterminal Angle Of 20 Times Pi Over 3 Question Mark
What Is A Coterminal Angle In Trigonometry
Coterminal Angle Calculator
What Is A Coterminal Angle In Trigonometry
How To Find Coterminal Angles
What Is A Coterminal Angle Example
How To Find Coterminal Angles In Radians
Negative Coterminal Angle
Coterminal Meaning
Coterminal And Reference Angles
What Is A Coterminal Angle